Construction of nilpotent Lie algebras with complex structures

Speaker: Adela Latorre (Polytechnic University of Madrid, Spain)

Time: September 21, 2023 17:00-18:00

Location: Zoom ID: 904 645 6677  Password:2023

Abstract: Complex manifolds can be characterized as pairs (M,J), where M is an even-dimensional differentiable manifold and J is a complex structure on it. Although the explicit construction of these J's is a difficult task, the problem can be slightly simplified when M is a nilmanifold and one restricts to the study of invariant complex structures on M. In this case, the problem of finding the pairs (M,J) is related to the classification of real nilpotent Lie algebras with complex structures. Such classification has been completed in real dimensions 4 and 6. However, the same techniques are difficult to apply when the dimension of the nilpotent Lie algebra is equal to or higher than 8. In this talk, we will present a new approach to the problem that will allow us to find every 8-dimensional real nilpotent Lie algebra having one-dimensional center and admitting complex structures.

Bio: Adela Latorre works at the Department of Applied Mathematics of the Polytechnic University of Madrid (Spain). Her main research area is complex non-Kähler geometry, although she is also interested in topics related to Lie algebras and Lie groups.

Previous lectures and talks:

Speaker: Dietrich Burde (University of Vienna, Austria)

Time: September 14, 2023 20:00-21:00

Location: Zoom ID: 904 645 6677  Password:2023

Abstract: Pre-Lie algebras and Post-Lie algebras arise in many areas of mathematics and physics. They are also related to etale affine representations of Lie algebras and algebraic groups. They also arise in the context of affine geometry on Lie groups, operad theory, deformation theory and Young-Baxter equations. For reductive groups, every etale affine representation is equivalent to a linear representation and we obtain a special case of a prehomogeneous representation. Such representations have been classified by Sato and Kimura in some cases. The induced representation on the Lie algebra level gives rise to a pre-Lie algebra structure on the associated Lie algebra. Pre-Lie algebra structures also correspond to left-invariant affine structures on Lie groups. In this talk we present results on the existence of etale affine representations of reductive groups and Lie algebras and discuss a related conjecture of V. Popov concerning flattenable groups and linearizable subgroups of the affine Cremona group.

Bio: Dietrich Burde is a professor at the Department of Mathematics at the University of Vienna. He obtained his Ph.D. in 1992 at the University of Bonn in Germany. His research interests lie in algebra and geometry, in the area of Lie groups, Lie algebras, algebraic groups and representation theory. He has received research grants from the Austrian Science Foundation on the topic "Affine Geometry on Lie Groups and Lie-algebraic Structures".

Semidirect products in digroups, skew braces, heaps, trusses, and in universal algebra

Speaker: Alberto Facchini (University of Padua)

Time: September 7, 2023 20:00-21:00

Location: Zoom ID: 904 645 6677  Password:2023

Abstract: We will present some algebraic structures that have recently received attention in view of their relation with set theoretic solutions of the Yang-Baxter equation. We will present the basic properties of digroups, skew braces, heaps, and trusses. In particular, we will focus on their semidirect products.

Bio: Alberto Facchini has been full professor of Algebra until 1999 at the University of Udine, and from 1999 to 2022 at the University of Padua. Now he is an Emeritus Professor. He has had six PhD students and has written more than 170 research papers published in mathematical journals, four textbooks, and two research books published by Birkhäuser Verlag (Basel). He is a member of Accademia San Marco and Accademia Galileiana di Scienze Lettere ed Arti in Padova. He has given scientific communications and lectures in almost 40 different countries. He has been one of the editors of a dozen mathematical journals, among which Bollettino dell'Unione Matematica Italiana, Communications in Algebra, Journal of Algebra and Its Applications, Journal of the Egyptian Mathematical Society, and Rendiconti del Seminario Matematico dell'Università di Padova. In Udine he has been dean of the Faculty of Science, in Padua he has been director of the Department of Pure and Applied Mathematics.

Introduction to Noncommutative Isolated Singularities and Resolutions

Speaker: Quanshui Wu (Fudan University)

Time: August 23, 2023 10:30-11:30

Location: 正新楼313室

Abstract: This will be an introductory talk to noncommutative isolated singularities and resolutions. I will recall some examples of commutative isolated singularities and noncommutative isolated singularities first. Then I will survey some related results, and introduce a version of noncommutative resolutions of commonly graded AS-Gorenstein singularities.

Bio: 吴泉水，复旦大学数学科学学院教授、博导、上海数学中心执行主任，主要从事非交换环论、非交换射影代数几何、Hopf代数的同调理论方面的研究。曾获教育部科技进步二等奖、教育部霍英东教育基金会青年教师奖、教育部高校“青年教师奖”、宝钢优秀教师奖、上海市优秀学术带头人、上海市优秀青年教师等，在Duke Math. J.、Trans. Amer. Math. Soc.、Israel J. Math.、J. Noncommut. Geom.、J. Algebra、J. Pure Appl. Algebra等国际著名期刊发表SCI论文50余篇，多次主持国家自然科学基金面上项目，曾担任SCI杂志Comm. Algebra编委。

Noncommutative moduli spaces

Speaker: Andrey Lazarev (Lancaster University)

Time: August 21-25, 2023

Location: 正新楼313室

Abstract: The aim of this minicourse is to outline the construction of global moduli spaces of different objects of algebraic and geometric nature (such as flat connections in vector bundles, modules over associative algebras, objects in dg categories etc.) in a homotopy invariant context. The first part of the course will explain how the local Koszul duality of Hinich and Keller-Lefevre provides a suitable context for studying local moduli problems (also known as deformations). The second part is devoted to the more recent work constructing the corresponding global theory. The global theory shares some properties with the local one but involves several significantly new features, most notably the use of dg categories. The emphasis will be placed on explaining the conceptual picture rather than on technical proofs. Various instructive examples will be given.

Bio: Andrey Lazarev is a professor of pure mathematics at the University of Lancaster. His recent research deals with the homotopy theory of differential graded algebras and categories, derived categories and higher phenomena. It has applications in rational homotopy theory, theory of operads and operadic algebras, algebraic topology and pure algebra. Andrey Lazarev is the Managing Editor of the journal《Bulletin of the London Mathematical Society》, as well as a Member of the Editorial Board of the journal《Higher Structures》.

 

授课日期 Date of Lecture	课程名称（讲座题目） Name (Title) of Lecture	授课时间 Duration  (Beijing Time)	参与人数 Number of Participants
Aug 21, 2023	Maurer-Cartan (MC) elements and gauge transformations. Deformation theory	10:30-11:30	30
Aug 21, 2023	Model categories and infinity categories, examples: dg algebras and dg Lie algebras	16:30-17:30	30
Aug 22, 2023	Coalgebras and Lie-commutative Koszul duality.	10:30-11:30	30
Aug 22, 2023	Representability of deformation functors	16:30-17:30	30
Aug 24, 2023	MC elements in dg associative algebras and associated dg categories. Homotopy  gauge equivalences	10:30-11:30	30
Aug 24, 2023	Homotopy theory of dg categories	16:30-17:30	30
Aug 25, 2023	Global Koszul duality as a Quillen equivalence between infinity-categories of dg algebras and dg coalgebras	10:30-11:30	30
Aug 25, 2023	Representability of noncommutative moduli space functors	16:30-17:30	30

 

On the volume of hyperbolic tetrahedron

Speaker: Nikolay Abrosimov (Sobolev Institute of Mathematics of RAS)

Time: August 20, 2023 10:30-11:30

Location: 正新楼313室

Abstract: The talk will give an overview of the latest results on finding exact formulas for calculating the volumes of hyperbolic tetrahedra. The classical formula of G. Sforza [1] expresses the volume of a hyperbolic tetrahedron of a general form in terms of dihedral angles. Its modern proof is proposed in [2]. The formula in terms of edge lengths is obtained in the recent joint work of the author with B. Vuong [3]. The known formulas for the volume of a hyperbolic tetrahedron of a general form are very complicated and cannot always be applied to calculate the volumes of more complex polyhedra. So, a natural question arises to find more convenient and simple formulas for sufficiently wide families of hyperbolic tetrahedra. At the end of the talk, we will consider hyperbolic tetrahedra of special types: ideal, biorthogonal, 3-orthogonal and their generalizations. The volume of the ideal and biorthogonal tetrahedron was known to N.I. Lobachevsky. We will present new formulas for calculating volumes and normalized volumes of a trirectangular hyperbolic tetrahedron [4] as well as its generalization for the 4-parameter family of tetrahedra with one edge orthogonal to the face. The latter formulas can be used to calculate the volumes of more complex polyhedra in the Lobachevsky space.

References:

[1] G. Sforza, Spazi metrico-proiettivi // Ricerche di Estensionimetria Integrale, Ser. III, VIII (Appendice), 1907, P. 41–66.

[2] N.V. Abrosimov, A.D. Mednykh, Volumes of polytopes in constant curvature spaces // Fields Inst. Commun., 2014, V. 70, P. 1–26. arXiv:1302.4919.

[3] N. Abrosimov, B. Vuong, Explicit volume formula for a hyperbolic tetrahedron in terms of edge lengths // Journal of Knot Theory and Its Ramifications, 2021, V. 30, No. 10, 2140007. arXiv:2107.03004.

[4] N. Abrosimov, S. Stepanishchev, The volume of a trirectangular hyperbolic tetrahedron // Siberian Electronic Mathematical Reports, 2023, V. 20, No. 1, P. 275–284.

Bio: Professor Nikolay Abrosimov is a professor at the Department of Mechanics-Mathmatics of Novosibirsk State University. He received a Candidate of Sciences in physics and mathematics in 2009 from the Sobolev Institute of Mathematics. His research interests are hyperbolic geometry, volumes of non-Euclidean polytopes, geometry and topology of three-dimensional manifolds, orbifolds, knots and links. He has more than 25 research publications. He is a chairman of the Council of Scientific Youth of the Institute of Mathematics of the Siberian Branch of the Russian Academy of Sciences.

Volumes of generalized hyperbolic polyhedra and hyperbolic links

Speaker: Andrei Vesnin (Sobolev Institute of Mathematics of RAS)

Time: August 20, 2023 09:30-10:30

Location: 正新楼313室

Abstract: A polyhedron in a three-dimensional hyperbolic space is said to be generalized if finite, ideal and truncated vertices are admitted. In virtue of Belletti's theorem (2021) the exact upper bound for volumes of generalized hyperbolic polyhedra with the same one-dimensional skeleton G is equal to the volume of an ideal right-angled hyperbolic polyhedron whose one-dimensional skeleton is the medial graph for G. We will present the upper bounds for the volume of an arbitrary generalized hyperbolic polyhedron, where the bonds linearly depend on the number of edges. Moreover, it is shown that the bounds can be improved if the polyhedron has triangular faces and trivalent vertices. As an application there are obtained new upper bounds for the volume of the complement to the hyperbolic link having more than eight twists in a diagram. The results under discussion are based on the preprint arXiv:2307.04543 (https://arxiv.org/abs/2307.04543).

Bio: Professor Andrei Vesnin is head of the Laboratory of Applied Analysis, Sobolev Institute of Mathematics and a professor of Geometry and Topology, Novosibirsk State University. He received a Candidate of Sciences in physics and mathematics in 1991 from the Sobolev Institute of Mathematics for the thesis “Discrete groups of reflections and three-dimensional manifolds”, and a Doctor of Sciences in physics in mathematics in 2005 for the thesis “Volumes and isometries of three-dimensional hyperbolic manifolds and orbifolds”.

    Professor Vesnin's research interests include low-dimensional topology, knot theory, hyperbolic geometry, combinatorial group theory, graph theory and applications. In 2008, Prof. Vesnin was elected as corresponding member of the Russian Academy of Sciences. He is a member of the editorial board of the journal "International Journal of Mathematics and Computer Science", and doctoral dissertation council at the Institute of Mathematics SB RAS.

 

Previous lectures and talks:

Yang-Baxter equation, Rota-Baxter operators and corresponding algebraic systems

Speaker：Valeriy Bardakov （Tomsk State University）

Time：2023年6月28日 14:30-15:30

Location：吉林大学数学楼3楼第5研讨室

Abstract: Yang-Baxter equation is a famous equation in mathematical physics, knot theory and braid theory. There are different generalization of this equation. In particular, tetrahedron equation and n-simplex equation. To describe solutions of these equations where introduced different algebraic systems: rack, quandle, skew brace and some other. The Yang-Baxter equation connects with Rota-Baxter operator on some algeras and groups. Im this talk we will speak on this things and connections between them.

Bio: Valeriy Bardakov is from Tomsk State University. He is a professor of department of algebra and mathematical logic, Faculty of Mechanic-Mathematics, Tomsk State University. His research interests are group theory, knot theory, braid group, automorphism group, symmetric group, PDE, multidimensional inverse problem, evolution equations and integral geometry.  He is an author of more than 100 publications.

Since 1995, he has been running the algebraic seminar, "Evariste Galois", at NSU. Since 2000, together with A. Yu. Vesnin, he has been teaching a special course "Algebraic Methods in Knot Theory" at Novosibirsk State University. In 1993, he won the prize M. I. Kargapolov for young mathematicians for solving problems of the Kourovskaya Notebook.

Simplicial structure on pure singular braid groups of camomile type

Speaker：Tatiana Kozlovskaia （Tomsk State University）

Time：2023年6月28日 13:30-14:30

Location：吉林大学数学楼3楼第5研讨室

Abstract: In my talk we recall some definitions from Knot Theory, Braid Theory and the construction of J. Wu and F. Cohen which connects braid groups and homotopy groups of 2-sphere.  In more detail we discuss singular braid groups and its subgroup of pure singular braid group. We describe presentation of these groups and linear representation.

Bio: Tatiana Kozlovskaia is from Scientific and Educational Mathematical Center of Tomsk State University. She is an associated professor of Department of Geometry, Faculty of Mechanic-mathematics, Tomsk State University. Her research interests are 3-dimensional topology, theory of 3-manifolds, low-dimensional geometry, Lens spaces, and Fundamental polyhedra.

Contact geometry via homogeneous symplectic geometry with applications

 

Speaker：Katarzyna Grabowska （University of Warsaw, Department of Physics）

Time：2023年5月24日 18:00-20:00

Meeting ID：ZOOM Id：904 645 6677，Password：2023

会议链接：

https://us02web.zoom.us/j/9046456677?pwd=UHErd3RJVzFsNzNnczFZYm9uYlV6QT09

Abstract: During the talk she will present the novel approach to contact geometry according to which contact structures are not `odd-dimensional generalizations’ of symplectic geometry but rather particular examples of symplectic geometry, namely homogeneous symplectic principal bundles (with an action of the multiplicative group of non-zero reals). In this setting we are able to construct contact Hamiltonian vector fields even if the global contact form does not exist on the contact manifold in question. The homogeneous symplectic language is also suitable for contact Hamilton-Jacobi theory and contact reductions.        

Bio: Katarzyna Grabowska works in the Department of Mathematical Methods in Physics at the Faculty of Physics. She is interested in differential geometric methods in physics and differential geometry in general.

 

Topological K-theory of discrete groups

Speaker：Bailing Wang （The Australian National University）

Time：2023年5月19日 10:30-11:30

Meeting ID：腾讯会议：516-539-518

Abstract:  In the 1980’s motivate by the Atiyah-Singer index formula,  Baum and Connes constructed a  topological K-theory of a discrete group  $\Gamma$, together with  an assembly map $\mu$  from this mysterious group to the K-theory group of the reduced  C^∗ -algebra of $\Gamma$.  They conjectured that this assembly map is an isomorphism.  The validity of this conjecture implies Novikov conjecture, Gromov-Lawson-Rosenberg conjecture and Kadison-Kaplansky conjecture.                     

The mathematical details of this construction and the well-definedness of the assembly map were somewhat missing in their original paper. I will briefly explain some of my earlier work with Paulo Carrillo Rouse on filling up these details, and some recent work with Paulo Carrillo Rouse and Hang Wang on an assembly map to periodic cyclic homology and the Chern-Connes pairing formula for any discrete group.

Bio: 王百灵，澳大利亚国立大学教授。1998 年 4月毕业于澳大利亚阿德莱德大学并获得博士学位。毕业先后在德国波恩马普所，法国高等科学研究所， 苏黎士大学做博士后和访问学者。2005年至今在澳大利亚国立大学工作。主要研究规范场理论在低维拓扑中的拓扑不变量、twisted K－同调和twisted指标理论、Gromov－Witten模空间和哈密尔顿Gromov－Witten模空间的K-理论等领域。

 

LIE GROUPOIDS IN INFORMATION GEOMETRY

 

Speaker：Janusz Grabowski  （Polish Academy of Sciences）

Time：2023年5月10日 15:50-16: 50

Meeting ID：ZOOM Id：904 645 6677，Password：2023

会议链接：

https://us02web.zoom.us/j/9046456677?pwd=UHErd3RJVzFsNzNnczFZYm9uYlV6QT09

Abstract:  After a general introduction to the information geometry, I will show that a natural general setting for statistical and information geometry is the one provided by Lie groupoids and Lie algebroids. The contrast functions are defined on Lie groupoids and give rise to a two-form and a three-form on the corresponding Lie algebroid. If the two-form is non-degenerate, it defines a pseudo-Riemannian metric on the Lie algebroid and a family of Lie algebroid torsion-free connections, including the Levi-Civita connection of the metric. In this framework, the standard two-point contrast functions are understood as functions on the pair groupoid MxM and generate a standard (pseudo-)Riemannian metrics on M, and families of affine connections on the Lie algebroid TM.

 

Bio: Professor Janusz Grabowski is the Head of the Department of Mathematical Physics and Differential Geometry in the Institute of Mathematics, Polish Academy of Sciences. His main interests are differential geometry and mathematical physics. As an author of about 140 scientific papers, he published fundamental results on Lie algebras of vector fields, diffeomorhism groups, Lie systems, Poisson and Jacobi manifolds, Lie groupoids and algebroids, Lagrangian and Hamiltonian mechanics (including mechanics on contact manifolds), supergeometry, geometry of quantum states and entanglement, etc. His personal page is https://www.impan.pl/~jagrab/.

 

 

Regular subgroups, skew braces, gamma functions and Rota–Baxter operators

 

Speaker：Andrea Caranti （University of Trento, Italy）

Time：2023年4月19日 20:00-22:00

Meeting ID：ZOOM Id：904 645 6677，Password：2023

会议链接：

https://us02web.zoom.us/j/9046456677?pwd=UHErd3RJVzFsNzNnczFZYm9uYlV6QT09

Abstract: Skew braces, a novel algebraic structure introduced only in 2015, have already spawned a sizeable literature. The skew braces with a given additive group structure correspond to the regular subgroups of the permutational holomorph of such a group. These regular subgroups can in turn be described in terms of certain so-called gamma functions from the group to its automorphism group, which are characterised by a functional equation. We will show how gamma functions can be used in studying skew braces, underlining in particular their relationship to Rota-Baxter operators.

Bio: Andrea Caranti is a Senior Professor of Algebra at the University of Trento, Italy. He has worked mainly in group theory (nilpotent groups, automorphisms, applications to cryptography) and on graded, modular Lie algebras. His recent work concerns group-theoretical aspects of skew braces.

On pseudo-Euclidean Lie algebras whose Levi-Civita product is left Leibniz

 

Speaker：Said Benayadi （University of Lorraine-Metz）

Time：2023年3月30日 20:00-21:00

Meeting ID：ZOOM Id：904 645 6677，Password：2023

会议链接：

https://us02web.zoom.us/j/9046456677?pwd=UHErd3RJVzFsNzNnczFZYm9uYlV6QT09

Abstract: We study a class of Lie algebras which contains the class of quadratic Lie algebras and the class of Milnor Lie algebras, namely, Lie algebras endowed a pseudo-Euclidean metric such its Levi- Civita product is left Leibniz. We call them Levi-Civita left Leibniz Lie algebras LCLL for short. We show that a Lie group (G, h) endowed with a left invariant pseudo-Riemannian such that the corresponding Lie algebra is LCLL is complete and locally symmetric. Moreover, we prove that any Euclidean LCLL Lie algebra is the product of quadratic a Lie algebra and a flat Euclidean Lie algebra. We develop an adapted version of the process of double extension to construct LCLL Lie algebras. We show that Lorentzian or flat LCLL Lie algebras can be obtained by this process.

Bio: Said Benayadi is a professor in University of Lorraine-Metz. His research interest is nonassociative algebras.

 

Singular foliations and Q-manifolds

 

Speaker：Camille Laurent-Gengoux （Université de Lorraine, Metz）

Time：2023年3月22日 16:00-17: 00

Meeting ID：ZOOM ID：904 645 6677，Password：2023

会议链接：

https://us02web.zoom.us/j/9046456677?pwd=UHErd3RJVzFsNzNnczFZYm9uYlV6QT09

Abstract: In this talk, we will explain how a Q-manifold (equivalently, a Lie infinity algebroid) can be associated to a singular foliation, and how this Q-manifold helps in studing the geometry of the latter. Joint works with Sylvain Lavau, Ruben Louis,  Leonid Ryvkin, Thomas Strobl.

Bio: Camille Laurent-Gengoux is a professor in Université de Lorraine, Metz. He mainly studies Poisson geometry and related structures. He authored more than 40 scientific articles in J. Eur. Math. Soc., Math. Ann., Adv. Math., Int. Math. Res. Not. and other journals.

 

On a certain family of vertex algebras associated with vertex algebroids

 

Speaker：Haisheng Li （Rutgers University-Camden）

Time：2023年3月9日 09:00-10:00

Meeting ID：ZOOM Id：904 645 6677，Password：2023

会议链接：

https://us02web.zoom.us/j/9046456677?pwd=UHErd3RJVzFsNzNnczFZYm9uYlV6QT09

Abstract: This talk is about a family of vertex algebras associated with vertex algebroids, constructed by Gorbounov, Malikov, and Schechtman. As the main result, we classify all the (graded) simple modules for such vertex algebras and we show that the equivalence classes of graded simple modules one-to-one correspond to the equivalence classes of simple modules for the Lie algebroids associated with the vertex algebroids. To achieve our goal, we construct and exploit a Lie algebra from a given vertex algebroid. This talk is based on a joint work with Gaywalee Yamskulna.

Bio: Haisheng Li is a professor of Rutgers University-Camden. His main research is on vertex operator algebras and quantum vertex algebras. Among the main results are conceptual constructions of vertex algebras and their modules, twisted modules; A theory of quasi modules; A theory of (weak) quantum vertex algebras and φ-coordinated modules; A conceptual association of double Yangians and quantum affine algebras with quantum vertex algebras. He published more than 100 articles in Duke Math. J., Com. Math. Phys., Adv. Math., Tans. AMS and other Internationally renowned journals.

 

On local integration of Lie brackets

Speaker：María Amelia Salazar Pinzón （Universidade Federal Fluminense）

Time：2023年2月16日 21:00-22:00

Meeting ID：ZOOM Id：904 645 6677，Password：2023

会议链接：

https://us02web.zoom.us/j/9046456677?pwd=UHErd3RJVzFsNzNnczFZYm9uYlV6QT09

Abstract: The foundation of Lie theory is Lie's three theorems that provide a construction of the Lie algebra associated to any Lie group; the converses of Lie's theorems provide an integration, i.e. a mechanism for constructing a Lie group out of a Lie algebra. The Lie theory for groupoids and algebroids has many analogous results to those for Lie groups and Lie algebras,however, it differs in important respects: one of these aspects is that there are Lie algebroids which do not admit any integration by a Lie groupoid. In joint work with Cabrera and Marcut, we showed that the non-integrability issue can be overcome by considering local Lie groupoids instead. In this talk I will explain a construction of a local Lie groupoid integrating a given Lie algebroid and I will point out the similarities with the classical theory for Lie groups and Lie algebras.

Bio: María Amelia is a professor at Departamento de Matemática Aplicada (GMA) of the Universidade Federal Fluminense (UFF), Brazil.The main research interests are Lie groupoids, Lie algebroids, Lie pseudogroups, geometry of PDE's, Poisson geometry, contact and symplectic geometry.

 

Local and 2-local derivations and automorphisms of Octonian algebras 

Speaker：Shavkat Ayupov (Institute of Mathematics, Uzbekistan Academy of Sciences)

Time：2023年2月9日 16:00-17:00

Meeting ID：ZOOM Id：904 645 6677，Password：2023

会议链接：

https://us02web.zoom.us/j/9046456677?pwd=UHErd3RJVzFsNzNnczFZYm9uYlV6QT09

Abstract:

The talk is devoted to description of local and 2-local derivations (respectively, automorphisms) on octonian algebras. We shall give a general form of local derivations on the octonion algebra O(F) over a field F with zero characteristic. This description implies that the space of all local derivations on O(F) when equipped with Lie bracket is isomorphic to the Lie algebra so7 O(F) of all real skew-symmetric 7 × 7-matrices over F. At the same time the Lie algebra of all derivations are isomorphic to the exceptional Lie algebra g₂(F). It follows that the octonion algebra O(F) and Malcev algebra M7(F) over the field F are simple non associative algebras which admit pure local derivations, that is, local derivations which are not derivation.

Further we consider 2-local derivations on the octonion algebra O(F) over an algebraically closed field F and prove that every 2-local derivation on O(F) is a derivation. But for the field R of real numbers 2-local derivations on the octonian algebra O(R) form a Lie algebra which is essentially larger than   the Lie algebra g₂(R) of derivations. we apply these results to problems for the simple 7-dimensional Malcev algebra. We shall give a general form of local automorphisms on the octonion algebra O(F). This description implies that the group of all local automorphisms on O(F) is isomorphic to the group O₇(F) of all orthogonal 7 × 7-matrices over F, and it is essentially larger than the group of all automorphisms.

We also consider 2-local automorphisms on the octonion algebra O(F) over an algebraically closed field F and prove that every 2-local automorphism on O(F) is an automorphism. At the same time the group of 2-local automorphisms of O(R) is larger than the group of automorphisms of O(R). As a corollary we obtain descriptions of local and 2-local automorphisms of seven dimensional simple Malcev algebra.

Bio: Shavkat Ayupov is the Director of V.I. Romanovskiy Institute of Mathematics, Uzbekistan Academy of Sciences. His field of scientific interest include Theory of Operator Algebras and Quantum Probability, Structure theory of Non-associative algebras (Jordan, Lie, Leibniz, etc.). He is the authors of several monograph devoted to Real and Jordan structures on Operator Algebras, also to the structure theory of Leibniz algebras.   Sh. Ayupov is a Member of Uzbekistan Academy of Sciences (since 1995), Fellow of TWAS (The World Academy of Sciences) (since 2003), Senior Associate of ICTP (International Centre for Theoretical Physics) (2008 – 2013), Guest Professor of Sichuan University (Chengdu, China) (2015-2021). He is the Managing Editor of Uzbek Mathematical Journal and editor of “Advances in Operator Theory”.

In 2017, he was awarded the State Prize of the first degree in the field of Science and Technology of the Republic of Uzbekistan.

Properties of polynomial maps and two-dimensional Jacobian conjecture

Speaker：Properties of polynomial maps and two-dimensional Jacobian conjecture

Time：2022年10月14日  9:30-10:30 Moscow time

Meeting ID：ZOOM ID：862 062 0549，Code：2022

会议链接：

https://us02web.zoom.us/j/8620620549?pwd=bGhsaG15WjRza2V3ZEN4TzJYZ1FZQT09

Abstract: For any two polynomials on two variables with a nonzero Jacobian determinant, there corresponds a polynomial map known as a Keller map. In this talk, the speaker will report 3 properties of Keller maps, which are used to give a proof of the two-dimensional Jacobian conjecture in the speaker’s paper entitled ``a proof of 2-dimensional Jacobian conjecture'' at arXiv:1603.01867.

Bio: Yucai Su is a professor at Tongji University and Jimei University. He has successively worked as a visiting scholar and postdoctoral researcher at Queen Mary and Westfield College, the University of London, Concordia University, and the University of Quebec at Montreal for 7 years. His main research interests include Lie algebras, representation theory and Jacobian problem. In particular, he has studied the Jacobian Conjecture for eighteen years.  He is an editor of Algebra Colloquium and Journal of Mathematical Study, and published over 100 papers in Adv. Math., J. Eur. Math. Soc., Proc. London Math. Soc., Comm. Math. Phys., Math. Z., Israel J. Math., etc.  Recently, using the local bijectivity of Keller maps, he solved the 2-dimensional Jacobian Conjecture.

 

FROM OPERADS TO OPERADIC CATEGORIES

Speaker：Michael Batanin （Institute of Mathematics of Czech Academy of Science, Charles University, Prague）

Time：2022年09月30日 16:30-17:30 

Meeting ID：ZOOM ID：862 062 0549，Code：2022

会议链接：

https://us02web.zoom.us/j/8620620549?pwd=bGhsaG15WjRza2V3ZEN4TzJYZ1FZQT09

Abstract: Operads were introduced at the end of 1960s by Boardman, Vogt and P.May as spaces parametrising multivariable operations and their substitutions. In the 1990's however, it was realised that there are other interesting types of operads such as cyclic and modular operads of Getzler and Kapranov (motivated by TQFT development) and globular n-operads of Batanin parametrising higher categorical compositional structures. In 2015 Batanin and Markl introduced operadic categories in order to develop a consistent theory of operadic like structures. In my talk I explain what operadic category and associated category of operads are and why this is a very rich and useful new algebraic concept.

Bio: The speaker graduated from Novosibisk State University in 1983. He is currently a Senior Researcher at the Institute of Mathematics of Czech Republic, and a Professor of Charles University in Prague. He is specialising in Algebraic Topology, Category Theory, Operads and related topics.

 

孤立波：1834-1984

Speaker：张大军 上海大学

Time：2022年09月23日 10:30-11:30 

Meeting ID：腾讯会议：309-266-962

腾讯会议链接：https://meeting.tencent.com/dm/jx2ianwNTLKT

Abstract: 这是一个关于“孤立波”的历史与发展的科普报告。1834年 John Scott Russell首次发现孤立波，1895年Korteweg和de Vries (KdV) 利用KdV方程描述了孤立波现象， 1965年 Kruskal 和 Zabusky在研究Fermi-Pasta-Ulam问题时发现并命名了“soliton” (孤立子), 两年以后Gardner-Greene-Kruskal-Miura 建立了反散射方法(Inverse Scattering Transform), 成为现代可积理论的起点。报告将回顾孤立波与可积系统在前150年(1834-1984)的历史，回顾期间(特别是1968-1984)出现的重要的可积模型以及重要的理论与方法进展。

Bio: 张大军，上海大学数学系教授，博士生导师。主要从事离散可积系统与数学物理的研究，包括离散可积系统的直接方法、多维相容性的应用、空间离散下的可积结构与连续对应等。曾访问Turku大学、Leeds大学、剑桥牛顿数学研究所、Sydney大学等学术机构。先后主持国家自然科学基金面上项目5项。目前担任离散可积系统国际系列会议SIDE (Symmetries and Integrability of Difference Equations)指导委员会委员(2012-)和国际期刊Journal of Physics A编委(2020-)。

Coarse geometry and operator algebras : a minicourse

 

Speaker：Yeong Chyuan Chung 博士后  Leiden University

Meeting ID：腾讯会议：869-2118-6000，密码：3721

腾讯会议链接：https://meeting.tencent.com/dm/x708itdk0Eq1

Abstract: Coarse geometry involves studying metric spaces by looking at them from far away, and it is important in areas of mathematics such as geometric group theory. In recent times, its interaction with operator algebras has also been an active area of research with applications to problems in topology and differential geometry. In this mini-course, we aim to introduce basic notions in coarse geometry and a class of operator algebras known as Roe algebras. Starting from coarse geometry, we will introduce basic definitions such as coarse embeddings and coarse equivalences, then we will introduce some coarse geometric invariants such as asymptotic dimension and property A. Next, we will introduce Banach algebras and C*-algebras with examples of these, then we will focus on Roe algebras and how they reflect the coarse geometry of the underlying metric spaces. Finally, we will briefly introduce operator K-theory and the coarse Baum-Connes conjecture involving the K-theory of Roe algebras.

 

授课日期 Date of Lecture	课程名称（讲座题目） Name (Title) of Lecture	授课时间 Duration	参与人数 Number of Participants
Sep 19, 2022	Introduction to coarse geometry: basic definitions and asymptotic dimension	19:30-20:30	30
Sep 20, 2022	Introduction to coarse geometry: property A	19:30-20:30	30
Sep 21, 2022	Banach algebras and C*-algebras with examples	19:30-20:30	30
Sep 22, 2022	Roe algebras I	19:30-20:30	30
Sep 26, 2022	Roe algebras II	19:30-20:30	30
Sep 27, 2022	Basic operator K-theory	19:30-20:30	30
Sep 28, 2022	K-theory of Roe algebras and the coarse Baum-Connes conjecture	19:30-20:30	30
Sep 29, 2022	A quick look at a counterexample to the coarse Baum-Connes conjecture	19:30-20:30	30

 

 

Bio: Yeong Chyuan Chung（钟永权），2017年博士毕业于美国德州农工大学, 主要从事粗几何、L^p算子代数、K-理论的研究。 近年来在J. Funct. Anal.，J. Noncommut. Geom.等杂志上发表多篇高水平论文。

 

 

Advanced Numerical Methods with Applications

 

Speaker：Andrey Dorogovtsev professor（Institute of Mathematics，NAS Ukraine）

Meeting ID：Zoom会议 ID：会议号：852 4434 8793，密码：075030

Abstract: This course aims to provide a solid introduction on some advanced numerical methods and its applications. The first part of the course will introduce the definition of local times and Gaussian functionals, then discuss some properties when local times as a Gaussian functionals. And the second part of the course is devoted to introduce Hausdorff measure and dimension, then use the Hausdorff measure and dimension to discuss sef-intersections set of Brownian motion. Professor Andrey will give students some time to understand the knowledge and give some small questions about the lecture.

授课日期 Date of Lecture	课程名称（讲座题目） Name (Title) of Lecture	授课时间 Duration (Beijing Time)	参与人数 Number of Participants
2022-09-19	Local times and sets of values of random fields	16:00-17:00	30
2022-09-21	Gaussian white noise and functionals from it	16:00-17:00	30
2022-09-26	Local times as a Gaussian functionals	16:00-17:00	30
2022-09-28	Local nondeterminism for Gaussian random fields	16:00-17:00	30
2022-10-03	Hausdorff measure and dimension	16:00-17:00	30
2022-10-05	Haussdorf dimension for sef-intersections set of Brownian motion	16:00-17:00	30

Bio: Andrey Dorogovtsev教授是乌克兰国家科学院通讯院士，乌克兰国家科学院数学所随机过程理论系主任，主要从事概率论及其相关领域研究，是乌克兰概率论研究方向学术领军人物之一。Andrey Dorogovtsev教授是乌克兰与德国、乌克兰与俄罗斯等国家联合项目的乌方负责人。同时，Andrey Dorogovtsev教授是《Theory of Stochastic Processes》、《Ukraine Mathematical Journal》等杂志的编委。

Noncommutative differential geometry and quantum effects of gravity

 

Speaker：张晓（中国科学院数学与系统科学研究院）

Time：2022年09月16日 10:30-11:30

Meeting ID：腾讯会议 ID：602-653-169

点击链接入会：https://meeting.tencent.com/dm/7TgVgfHRMj51

Abstract: We provide a rigorous theory of noncommutative metrics and curvatures in frame of deformation quantization. In terms of them, we are able to propose the noncommutative Einstein field equations. We show that the deformation of classical pp-waves are exact solutions of vacuum field equations. We also find that the quantization of spherically symmetric metrics is renormalizable and the deformation of classical Schwarzschild solution is the quantum black hole solution which does not depend on time and can not be evaporated. The talk is based on the early joint works with Chaichian, Tureanu, D. Wang, R.B. Zhang as well as H. Gao recently.

Bio: 张晓，中国科学院数学与系统科学研究院数学研究所研究员，国家杰出青年基金及中科院百人计划获得者。现任广西大学君武学者和广西八桂学者，广西数学研究中心执行主任。从事广义相对论的数学研究，在广义相对论正能量问题及引力量子化的非交换几何理论上作出了系统性的贡献。

 

Higher algebraic structures-a minicourse

 

Speaker：Camilo Andres Angulo Santacruz（Universidade Federal Fluminense）

Meeting ID：ZOOM ID：862 062 0549   Password：2022

点击链接入会：

https://zoom.us/j/8620620549?pwd=bGhsaG15WjRza2V3ZEN4TzJYZ1FZQT09

Course Abstract：“Higher structure” is a term used loosely to refer to a large collection of structures that share a common theme. Suppose a mathematical structure consists of a collection of sets, functions between them, and some equations that the latter shall verify. Think of group structures, for instance, as the sets G, GxG, and the singleton, together with three functions (the multiplication, the unit, and the inverse) that verify the usual axioms of a group, which are equations relating them(!). The common idea behind higher structures is that they roughly are like mathematical structures, but replacing sets by homotopy types, equations by homotopies, and adding higher-order homotopies to enforce coherence.

Higher structures have an intricate history and abound in mathematics. We will focus on three types of higher structures that have algebraic flavor.In what follows we describe the plan of the lectures. We will start by giving a bit of a panoramic perspective on the history and emergence of the higher structures we will consider. After going through some preliminaries, we proceed to study them one by one, starting with the so-called L-infinity algebras, continuing with stacky groupoids, and closing with Lie n-groupoids. In each module, we go through definitions, examples, and main constructions. We conclude by trying and relating these structures among them and by giving an outlook for the directions in which these generalize by touching upon some recent research topics.

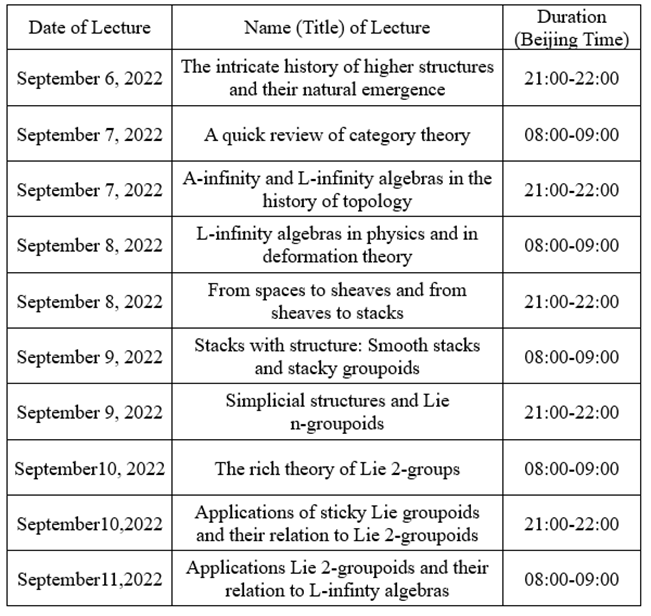
Bio：Camilo Andres Angulo Santacruz is a post doctor from Universidade Federal Fluminense, Brazil. He mainly study Poisson geometry and higher structures.

 

怪波/畸形波简介

 

Speaker：闫振亚（中科院数学院系统所）

Time：2022年09月09日 10:30-11:30

Meeting ID：腾讯会议 ID：737-700-086

点击链接入会：https://meeting.tencent.com/dm/VWCgdy2stinj

 

Abstract: 不同于通常的孤子(soliton), 怪波（rogue wave/rogon）拥有巨大的能量，也被称为畸形波、巨波、极端波等。它最早出现在深水海洋中，后来人们发现很多自然科学中也存在怪波现象，如光学、Bose-Einstein凝聚态、等离子体物理、大气科学、生物学和金融市场等。从某种意义来说，社会科学中的突发问题也属于怪波范畴。本报告介绍线性和非线性科学问题中怪波的背景、发展和挑战等。另外讨论怪波产生的一些物理机理等。

Bio: 闫振亚，中科院数学院系统所研究员，主要研究可积系统理论、怪波、PT对称、智能数学物理及交叉应用等。2019年获得国家自然科学基金杰出青年基金。

 

Operators on the universal enveloping algebras and quantisation of the argument shift method

 

Speaker：Georgy Sharygin（Lomonosov Moscow State University）

Time：2022年09月09日 21:00-22:00

Meeting ID：ZOOM ID：862 062 0549   Password：2022

点击链接入会：

https://zoom.us/j/8620620549?pwd=bGhsaG15WjRza2V3ZEN4TzJYZ1FZQT09

Abstract:  Argument shift method is an important and simple method to generate large commutative subalgebras in Poisson algebras, in particular in the algebras of functions on coadjoint representation of a Lie algebra. In the last 20 years a considerable in finding "quantized version" of such algebras was achieved: in the papers of Rybnikov, Molev and others one can find many examples of commutative subalgebras in the universal enveloping algebras of different Lie algebras, that "raise" the subalgebras, obtained by the argument shift method. However these subalgebras are constructed by "quantizing" concrete sets of generators in the "classical" argument shift subalgebras, and no general construction of "shifting" in the universal enveloping algebras is known. In my talk I will discuss a potential "quantum counterpart" of the shift in a particular case of the Lie algebra gl_n. It is based on the use of "quasi-derivations" of Ugl_n, introduced by Gourevitch and Saponov. I will describe these operators and discuss their relation with other constructions. I will also discuss the experimental data that supports the conjecture that one can define the quantum shift of the argument using these operators.  

Bio: Georgy Sharygin got his PhD from Moscow State University in 2000, and since that time he has been teaching Mathematics at all levels from High school to the PhD programs. He was invited speaker at many international conferences, was many times invited researcher in various international institutes. His research interests include deformation quantisation, non commutative geometry, topology, differential geometry and integrable systems.

 

The category of 2D rational CFT's

 

Speaker：孔良（南方科技大学）

Time：2022年09月02日 21:00-22:00

Meeting ID：腾讯会议 ID：939-656-494

点击链接入会：https://meeting.tencent.com/dm/aTqabg1ACJK5

Abstract:  In this talk, I will review the categorical study of 2D rational CFT's and topological defects in the last two decades. In the end, I will show how to use it to describe the category of 2D rational CFT's.  

Bio: 孔良，南方科技大学深圳量子科学与工程研究院研究员。1994年于中国科学技术大学获得物理学士学位，2005年于美国Rutgers，the State University of New Jersey获得数学博士学位。主要研究拓扑量子场论和共形场论的数学理论，以及在拓扑物质态中的应用。

 

可积系统中的Darboux变换简介

 

Speaker：刘青平（中国矿业大学）

Time：2022年08月19日 10:00-11:00

Meeting ID：腾讯会议 ID：721-493-311                           

点击链接入会：https://meeting.tencent.com/dm/BnT3kqNexgYQ

 

Abstract:  这是关于Darboux变换的一个科普报告。我们将从Jean Gaston Darboux发表于1882年一篇短文谈起，介绍什么是Darboux变换、构造Darboux变换的方法以及它在可积系统理论中的应用。

Bio: 刘青平，国务院政府特殊津贴获得者，北京市高等学校教学名师，1992年获英国Leeds大学博士学位，曾在中国科学院理论物理研究所和西班牙Complutense大学做博士后，现为中国矿业大学（北京）教授。从事可积系统理论及其应用研究。更多信息见个人主页：https://lxy.cumtb.edu.cn/info/1067/1238.htm

 

Morse指标定理简介

 

Speaker：胡锡俊（山东大学）

Time：2022年08月12日 10:00-12: 00

Meeting ID：腾讯会议 ID：324-276-209

点击链接入会：https://meeting.tencent.com/dm/syKSSjFXHH5R

Abstract:  Morse指标定理源于对测地线的研究，它将Morse指标表为测地线共轭点的重数之和。在本次演讲中我们将回顾Morse指标定理的经典结论并介绍最新的进展。 

Bio: 胡锡俊，山东大学教授。1997年7月和1999年7月在吉林大学分别获得学士学位和硕士学位，2002年7月在南开大学获得博士学位。2014年获得国家杰出青年基金，主要研究方向为哈密顿系统与非线性分析。在天体力学中周期解的稳定性问题，哈密顿系统指标理论及一类反应-扩散方程行波解的研究中做出了创新性的工作。

 

Symmetries: from groups to tensor categories

 

Speaker：Alexei Davydov（Ohio University）

Time：2022年08月12日 16:00-17: 00

Meeting ID：ZOOM ID：862 062 0549， Password：2022

点击链接入会：

https://us02web.zoom.us/j/8620620549?pwd=bGhsaG15WjRza2V3ZEN4TzJYZ1FZQT09

Abstract: Groups are mathematical ways of talking about symmetries. From its beginning Group Theory was driven by the idea of symmetry and its applications (e.g. Galois' proof of unsolvability of a quintic). One of the most spectacular applications of Group Theory was the classification of crystals done at the end of the 19th century. In the middle of the 20th century Group Theory was used to describe all known states of matter. Experimental developments of the condensed matter physics at the end of the 20th century were not fitting the standard Group Theory scheme. They forced us to generalize the mathematical formulation of symmetry. The talk will be about this generalized notion, the one of tensor category.  ​

Bio: Alexei Davydov is a Professor of Department of Mathematics, Ohio University. He mainly works on category theory and homological algebra.

Gravity properad, moduli spaces M_g,n, and string topology

Speaker：Sergei Merkulov   University of Luxembourg

Time：2022年08月05日 16:00-18: 00

Meeting ID：ZOOM ID：862 062 0549

Password：2022

点击链接入会：

https://us02web.zoom.us/j/8620620549?pwd=bGhsaG15WjRza2V3ZEN4TzJYZ1FZQT09

Abstract: ​Using Thomas Willwacher’s twisting endofunctor, and Kevin Costello’s theory of partially compactified moduli spaces of algebraic curves of arbitrary genus with marked points, we introduce a new dg properad which contains Ezra Getzler’s operad controlling genus zero moduli spaces. We discuss its applications in the theory of moduli spaces M_g,n, and in string topology.  

Bio: Sergei Merkulov is a Professor at the University of Luxembourg. He worked previously at the Russian Academy of Sciences, the Glasgow University (UK) and the Stockholm University (Sweden). He works on category theory, homological algebra and Differential geometry.

Thick morphisms of supermanifolds and homotopy algebras

Speaker：Theodore Voronov   University of Manchester

Time：2022年07月29日 16:00-18: 00

Meeting ID：ZOOM ID：862 062 0549

password：2022

点击链接入会：

https://us02web.zoom.us/j/8620620549?pwd=bGhsaG15WjRza2V3ZEN4TzJYZ1FZQT09

Abstract: Supergeometry can be used as a unifying language for many algebraic and differential-geometric constructions. Particular role here is played by homological vector fields, i.e. odd vector fields Q satisfying Q^2=0. They can be useful for describing “higher” or “homotopy” analogs of Poisson brackets. Recently I discovered a generalization of the notion of a smooth map (called by me “thick morphisms”) giving NONLINEAR pullbacks on smooth functions. “Thick morphisms” can in particular provide L_infinity morphisms for homotopy Poisson structures. There are other interesting connections, e.g. with Fourier integral operators. Thick morphisms also provide a nonlinear analog of classical functional-algebraic duality.   

Bio: Dr Theodore Voronov is a Professor in Pure Mathematics at the University of Manchester (UK). His research interests are on the crossroads of algebra, differential geometry, topology and mathematical physics; in particular, geometry of supermanifolds and its applications. See more at 

http://personalpages.manchester.ac.uk/staff/theodore.voronov.

 

 

 

Representations of the Fermion-Virasoro algebras 

Speaker：赵开明 Wilfrid Laurier University

 

Time：2022年07月15日 09:00-11:00

Meeting ID：

腾讯会议 ID：635-613-814

点击链接入会，或添加至会议列表：

https://meeting.tencent.com/dm/aEOWDPUh24oM

 

 

Abstract: We introduce Fermion algebras F and the Fermion-Virasoro algebras. They are infinite-dimensional Lie superalgebras. The progress on simple smooth modules and simple Harish-Chandra modules over these algebras will be discussed.

Bio: 赵开明，加拿大Wilfrid Laurier大学教授，主要研究方向为李代数。主持多项国家自然科学基金, 及加拿大NSERC基金。他在 Adv. Math., Tran. AMS, J. Lond. Math. Soc., J. Algebra 等国际刊物上发表学术论文100多篇。

Rota-Baxter bisystems and mixed bialgebras 

 

Speaker：Abdenacer Makhlouf（Université de Haute Alsace）

Time：2022年07月08日 16:00-17:00

Meeting ID：

ZOOM: 862 062 0549，Password: 2022

To Join Zoom Meeting:

https://us02web.zoom.us/j/8620620549?pwd=bGhsaG15WjRza2V3ZEN4TzJYZ1FZQT09

Abstract: In this talk, we deal with a generalization of the concept of Rota-Baxter operators defined by T. Brzezi´nski and called Rota-Baxter systems which appeared in [J. Algebra 460(2016):1- 25]. We provide a dual version and then consider a generalization to bialgebras, we introduce the notion of Rota-Baxter bisystem and construct various examples. On the other hand, we introduce a new type of bialgebras (named mixed bialgebras) which are consisting of an associative algebra and a coassociative coalgebra satisfying the compatible condition determined by two coderivations. We investigate coquasitriangular mixed bialgebras and the particular case of coquasitriangular infinitesimal bialgebras, where we give the double construction. This is a joint work with Tianshui Ma and Sergei Silvestrov.

Bio: Abdenacer Makhlouf is a Professor and the head of Mathematics Department at University of Haute Alsace, Mulhouse, France. His research covers structure, representation theory, deformation theory and cohomology of various types of algebras, including Nonassociative algebras, Hopf algebras and n-ary algebras.

 

Kahler-Ricci flow on smooth minimal models 

 

Speaker：张振雷（首都师范大学）

Time：2022年07月01日 10:00-12:00

Meeting ID：腾讯会议 ID：780-765-137

To Join Tencent Meeting: https://meeting.tencent.com/dm/5cjuobQFgOpb 

Abstract: It is a survey talk on the long-time behavior of a Kahler-Ricci flow on a smooth manifold with a semi-ample canonical line bundle.

Bio：张振雷，首都师范大学数学科学学院教授。2003年本科毕业于吉林大学数学科学学院；2008年博士毕业于南开大学陈省身数学研究所，导师方复全教授。2018年获国家自然科学基金杰出青年科学基金资助，主要研究Ricci流、Kahler-Ricci流。

 

奇妙的基础数学

Speaker：徐晓平（中国科学院大学）

Time：2022年06月24日 14:00-16:00

Meeting ID：腾讯会议 ID：703-956-224

To Join Tencent Meeting: https://meeting.tencent.com/dm/gtlAO1KvxQLo

Abstract：这是一个科普型报告。我们从“什么是数学”谈起。然后是：素数的故事，拉马驽金的故事，分隔函数，KdV方程，量子物理的基本数学模型。之后，我们讲：数学的残缺美，一些挑战性问题以及本人的一些研究体验。

Bio：徐晓平，中国科学院大学教授，中国科学院数学研究所所长。1992年在美国Rutgers大学取得博士学位，师从著名的李理论专家James Lepowsky 和Robert Lee Wilson。之后在香港科技大学工作十年。2002年回到中国科学院数学研究所工作， 2014年开始兼任中国科学院大学教授，自2022年3月起，任中国科学院数学研究所所长。获宝钢优秀教师奖。他在李代数、顶点算子代数（共形场论）和偏微分方程的代数解法等相关领域的研究中做出了重要贡献。

 

低维拓扑学的世纪回顾

Speaker：雷逢春（大连理工大学）

Time：2022年06月24日 10:00-12:00

Meeting ID：685-963-603

To Join Tencent Meeting: https://meeting.tencent.com/dm/49Yn9DtKVB4q

Abstract：拓扑学是当代核心数学的一个重要前沿领域，其渊源可追溯到欧拉早期的工作（1836年，哥尼斯堡七桥问题的解答）。拓扑学奠基于19世纪末, 在20世纪取得了梦幻般的发展, 进入21世纪更加兴旺发达。本报告将对拓扑学的发展作一个跨越世纪的通俗概要浏览, 重点介绍低维拓扑学的发展，即二、三和四维流形拓扑学的主要成就。

Bio：雷逢春，1990年12月博士毕业于吉林大学基础数学专业，研究方向为低维拓扑，现为大连理工大学数学科学学院教授、博士生导师。长期从事三维流形拓扑方面的研究工作，多次承担国家自然科学基金面上项目、重点项目和海外及港澳学者合作研究基金(延续)项目的研究工作。曾于1997年荣获国家教委科技进步二等奖(排名3)，2001年获黑龙江省杰出青年科学基金，2002年入选教育部“跨世纪优秀人才培养计划”。现为中国数学会常务理事，辽宁省数学会副理事长，大连市数学学会理事长。

 

Factorization of Shapovalov elements 

Speaker：Andrey Mudrov（Moscow Institute of Physics and Technology and University of Leicester）

Time：2022年06月17日 15:00-17: 00

Meeting ID：ZOOM ID：862 062 0549, Password:2022

Abstract：A classical result of J. Bernstein, I. Gelfand and S. Gelfand says that a singular vector in a Verma module over a simple complex Lie algebra can be obtained from its highest vector by applying a product of special elements of the negative nilpotent subalgebra called Shapovalov elements. We provide explicit formulas for those elements, and hence for singular vectors of the Verma modules, expressing them through certain matrix elements of the inverse contravariant Shapovalov form.

Bio：The speaker is currently an Associate Professor and a Senior Researcher at the Center of Fundamental Mathematics in MIPT, and an Honorary Lecturer at the University of Leicester. He is specializing in quantum groups, deformation quantization and related topics. 

 

关于最对称的双曲环面上的闭测地线

Speaker：张影（苏州大学）

Time：2022年06月10日 10:00-12:00

Meeting ID：564-626-700 

To Join Tencent Meeting：https://meeting.tencent.com/dm/p7VBNfhblItm

Abstract：在与李祥飞的合作工作中，我们研究具有最大对称性的完备的双曲环面上的闭测地线的性质，证明一般闭测地线的迹多项式的正性，一些简单闭测地线长度的单调性，并提出简单闭测地线的迹的凸性猜测，以及一般闭测地线的迹多项式系数的对数凹性猜测。

Bio：张影，1985.9-1999.5 吉林大学数学系本研学习、任教，1999.5-2004.7 新加坡国立大学读研，2006.3-2007.2 巴西国家数学所(IMPA)博士后，2009.6至今，苏州大学数学科学学院教授。从事几何拓扑学研究。

 

同调论简史 

Speaker：段海豹 (中国科学院数学与系统科学研究院)

Time：2022年5月27日 10:00-12:00

Meeting ID：899-680-387

To Join Tencent Meeting：meeting.tencent.com/dm/Il9TkCVA4hAL

Abstract：同调论是20世纪数学所诞生的一项新技术，是当代几何、拓扑、分析学的有力工具。报告通过 Riemann, Poincare, Brouwer, Hopf 等开拓者们的相关工作介绍，回顾同调论的背景、起源、以及发展历程。

Bio：段海豹，中国科学院数学与系统科学研究院研究员，1977年3月－1985年1月，在吉林大学数学系学习，获理学学士、硕士学位；1987年7月，在北京大学获博士学位。从事代数拓扑，微分拓扑和代数几何的教学、研究工作。 他在2005年获中国数学会陈省身数学奖，2010年获国家自然科学二等奖。

 

Lie 2-algebras from geometric structures 

Speaker：Zhangju LIU

Time：2022.5.13 14:00-16:00

Meeting ID：347-371-151

To Join Tencent Meeting：https://meeting.tencent.com/dm/rx9v5zMU7xnV

Abstract：The notion of Lie 2-algebras is introduced as categorification of Lie algebras, Which is one of the fundamental objects in higher Lie theory and has close connection with strongly homotopy Lie algebras. The Lie 2-algebra structure has enjoyed significant applications in both geometry and mathematical physics. Strict Lie 2-algebras are equivalent to Lie algebra crossed modules, which are classified by the third cohomology of a Lie algebra.

In this talk, we’ll review several Lie 2-algebras that come from geometric structures, namely, 2-plectic manifolds; Courant algebroids; homotopy Poisson manifolds and affine structures on Lie groupoids. A 2-plectic structure on a manifold is a nondegenerate closed 3-form. There is a Lie 2-algebra structure on functions and Hamiltonian 1-forms of a 2-plectic manifold A Courant algebroid is a vector bundle together with a bilinear form, a skew-symmetric bracket and an anchor map. The bracket satisfies the Jacobi identity up to a coboundary, which generates a Lie 2-algebra on the section space of the bundle and functions on the base manifold. Parallel to the fact that there is a one-to-one correspondence between Lie algebra structures on a vector space and linear Poisson structures on the dual space, there is a one-to-one correspondence between Lie 2-algebra structures on a 2-vector space and linear homotopy Poisson structures on the dual 2-vector space. On a Lie groupoid, vector fields that are compatible with the groupoid multiplication are called multiplicative. Multiplicative vector fields with the Schouten bracket form a Lie algebra, which is not invariant under the Morita equivalence of Lie groupoids. To define vector fields on a differentiable stack, one needs to extend the Lie algebra to a Lie 2-algebra formed by affine vector fields on a Lie groupoid, which is Morita invariant.

Bio：刘张炬，北京大学/河南大学教授，1982年1月本科毕业于吉林大学，1986年6月在北京大学获得博士学位。1999年获得国家杰出青年基金。主要从事数学物理、Poisson几何等领域的研究。曾入选教育部跨世纪优秀人才支持计划，2004年获得教育部自然科学一等奖。

 

Morse matching method for conformal cohomology 

Speaker：Pavel Kolesnikov (Sobolev Institute of Mathematics)

Time：2022.5.6 10:00-12:00

Meeting ID：862 062 0549

Password：2022

To Join Zoom Meeting：https://us02web.zoom.us/j/8620620549?pwd=bGhsaG15WjRza2V3ZEN4TzJYZ1FZQT09

Abstract：We will observe the matching method in the algebraic discrete Morse theory which provides us a powerful tool for finding Anick resolutions for associative algebras defined by generators and relations. We apply this method to find reduced Hochschild cohomologies of associative conformal algebras in order to study their relations with the cohomologies of Lie conformal algebras. In particular, we evaluate the Hochschild cohomology groups for the universal associative envelope U(3) of the Virasoro Lie conformal algebra.

Bio：Pavel Kolesnikov got his PhD in 2002 in Novosibirsk State University (NSU) and joined Sobolev Institute of Mathematics. Now he is a research fellow of the Institute and professor in the NSU. His main area of research interests is in the structure and combinatorial theory of associative and non-associative algebras, conformal and vertex algebras.

 

On the cobar-construction for non-simply connected spaces

Speaker：Andrey Lazarev ( Lancaster University )

Time：2022.04.22 16:00-18:00

Meeting ID：862 062 0549

Password：2022

To Join Zoom Meeting：https://us02web.zoom.us/j/8620620549?pwd=bGhsaG15WjRza2V3ZEN4TzJYZ1FZQT09

Abstract：The cobar-construction produces a differential graded (dg) algebra out of a dg coalgebra. When applied to the coalgebra of simplicial chains on a simply-connected space X, the resulting dg algebra models the chain algebra on the based loop space of X. This is a classical result of Adams and it has been known for over 60 years. In a recent breakthrough development, American mathematicians Rivera and Zeinalian removed the simple-connectivity assumption.  In this talk, I will explain Rivera-Zeinalian’s result, discuss its generalizations and connections with infinity-categories.

Bio：Andrey Lazarev is a professor of pure mathematics in the University of Lancaster. His recent research deals with homotopy theory of differential graded algebras and categories, derived categories and higher phenomena. It has applications in rational homotopy theory, theory of operads and operadic algebras, algebraic topology and pure algebra. Andrey Lazarev is the Managing Editor of the journal《Bulletin of the London Mathematical Society》， as well as a Member of the Editorial Board of  the journal《Higher_Structures》.

 

Boson-Fermion Correspondence and Its Applications to Integrable Hierarchies Revisited From The Point of View of Representation Theory and Random Walks

Speaker：Jian Zhou

Time：2022.04.15 10:00-12:00

Meeting ID：694-475-243

To Join Tencent Meetinghttps://meeting.tencent.com/dm/dbl4pDJLd3zS

Abstract：We revisit the boson-fermion correspondence and its applications to integrable hierarchies via representation theory of symmetric groups. This makes it natural to consider random walks on various diagrams and graphs related to symmetric groups. Random partitions, hypergeometric tau-functions and weighted Hurwitz numbers are then brought together under a unified probabilistic treatment, rooted in their connections to the fermionic Fock space. Various approaches to the representation theory of symmetric groups all turn out to be useful in this treatment. They include: the new approach of Okounkov and Vershik, the Hopf algebra approach of Zelevinsky, and the lambda-ring approach of Knutson. A connection to the interpolating statistics in the study of fractional quantum Hall effect will also be explained.

Bio：周坚，清华大学数学科学系教授，2005年国家杰出青年基金获得者、2009年入选国家“百千万人才工程”。他的研究领域为黎曼面的模空间与霍奇积分，拓扑场论，微分几何，弦理论等。周坚教授通过对超弦理论中Vafa学派的工作中出现的一些数学问题的研究，揭示了一些不同的数学分支之间的内在联系，他与合作者完成的“Marino-Vafa猜想的证明”入选2004年度“中国高校十大科技进展”。

 

Heegaard splitting: a survey

Speaker: 邱瑞锋 (华东师范大学)

Time：2022年04月08日 10:00-12:00

Meeting：#腾讯会议：680-593-921

https://meeting.tencent.com/dm/lxemeq0TOyx1

Abstract: Heegaard分解是紧致三维流形上普遍存在的组合拓扑结构，在三维流形理论的研究中起到了重要的作用。吉林大学是我国最早从事这一领域研究的研究群体，并直接或间接地培养了一批这一领域的专家学者。这个报告将介绍这一领域的国内外发展历史及现状。

Bio：邱瑞锋，华东师范大学教授，主要从事三维流形及纽结理论的研究，代表性工作有：（1）证明了Heegaard分解理论中的Gordon猜想，（2）构造了纽结洞数理论中的Morimoto猜想的反例等。

 

Orbifold theory and modular extensions
 

Speaker: 董崇英 美国加州大学Santa Cruz 分校

Time：2022-03-25 10:00-12:00 

Abstract: Orbifold theory studies a vertex operator algebra V under the action of a finite automorphism group G.  The main objective is to understand the module category of fixed point vertex operator subalgebra V^G.  We prove a conjecture by Dijkgraaf-Pasquier-Roche on V^G- module category if V is holomorphic. We also establish a connection between rational orbifold theory and minimal modular extensions. Our work is based on the previous results on modular extensions by Drinfeld-Gelaki-Nikshych-Ostrik and Lan-Kong-Wen. This is a joint work with Richard Ng and Li Ren.

Bio: 董崇英，美国加州大学Santa Cruz分校终身教授，国际上无限维李代数和顶点算子代数领域最杰出的数学家之一,多年来一直从事无穷维李代数、顶点算子代数、Orbifold理论以及广义月光等方面的研究。在Acta Math.、Duke Math. J.、Adv. Math.、Comm. Math. Phys.等国际著名期刊发表论文100多篇，总引用超过3000次，其中包括菲尔兹奖获得者Drinfeld、Zelmanov和Borcherds以及著名数学家如Beilinson和Kac等人的重要引用。主持多项美国自然科学基金，并担任杂志Algebra Colloquium的主编以及Science China Mathematics等多个杂志的编委。
Meeting：128-767-072加入会议：
https://meeting.tencent.com/dm/hyMIOAPeEsWf

Deformations of Symplectic Foliations

Speaker: Marco Zambon (KU Leuven)

Time: 2022.03.04  20:00-22:00

Location:

           To Join Zoom Meeting: 

           https://us02web.zoom.us/j/8620620549?pwd=bGhsaG15WjRza2V3ZEN4TzJYZ1FZQT09

          Meeting ID：862 062 0549

          Password：2022

Abstract:

Symplectic foliations and regular Poisson structures are the same thing. Taking the latter point of view, we exhibit an algebraic structure that governs the deformations of symplectic foliations, i.e. which allows to describe the space of symplectic foliations nearby a given one.  Using this, we will address the question of when it is possible to prolong a first order deformation to a smooth path of symplectic foliations. We will be especially interested in the relation to the underlying foliation. This is joint work with Stephane Geudens and Alfonso Tortorella.

Bio:

Marco Zambon is an associate professor at KU Leuven, Belgium. He works on topics related to Poisson geometry, in particular on those related to foliations, deformations and Lie theory.

 

 

 

Diophantine approximations

 

Speaker：Nikolay Moshchevitin (Moscow State University)

Location：Zoom

          ID：894 9789 1576

         Password：237198

Lecture1. Continued fractions.
2022.01.19， 13：30-15：00

Representation of real numbers as continued fractions. Approximation by convergents, Perron's formula.
Irrationality measure function.
Lagrange and Dirichlet spectra. Minkowski diagonal continued fraction and the related spectrum. Geometry of continued fractions algorithm.


Lecture2. Distribution of Farey fractions.
2022.01.20， 13：30-15：00

Elements of basic number theory. Euler and Moebius functions.
Integral representation of greatest common divisor. Counting the number of reduced fractions in [0,1].
Fraenel's theorem. Relation to Riemann zeta-function.


Lecture3. Farey tree and Minkowski function.
2022.01.26， 13：30-15：00

Stern-Brocot sequences. Minkowski question-mark function.
Salem's theorem on the derivative. Fixed points problem. An analog to Fraenel's theorem.
Fourier-Stieltjes coefficients.


Lecture4.Multidimensional approximation.
2022.01.27， 13：30-15：00

Simultaneous approximation to real numbers. Linear forms close to xero.
Basic laws of approximation. Best approximations and phenomenon of degeneracy of dimension.
Diophantine exponents and Jarnik's inequalities.

 

Bio：Professor Nikolay Moshchevitin is working at Moscow State University. His research interests include geometry of numbers, geometric theory of Diophantine approximations, ergodic and combinatorial theory of numbers, theory of dynamical systems. He has published more than 70 papers with more than 400 citations. He was awarded the State Prize of the Russian Federation in 1998.

 

Geometry of Gaussian random curves

Speaker: Andrey Dorogovtsev (Institute of Mathematics，NAS Ukraine)

Time: January 4, 2022 - February 22, 2022

Location: Zoom

          Meeting ID: 858 7772 7123

          Password: 803617

Abstract:

This course aims to provide a solid introduction on the geometry of Gaussian random curves. Since the theory of geometry of Gaussian random curves is based on the concept of Gaussian random process and fields, the first part of the course will be devoted to some properties of this process and fields. And the second part of the course is devoted to discussion of geometry of Gaussian random curves like the Self-intersection local times for planar Brownian motion and the hitting probabilities for planar Broownian motion. Professor Andrey will give students some time to understand the knowledge and give some small questions about the lecture.

Schedule:

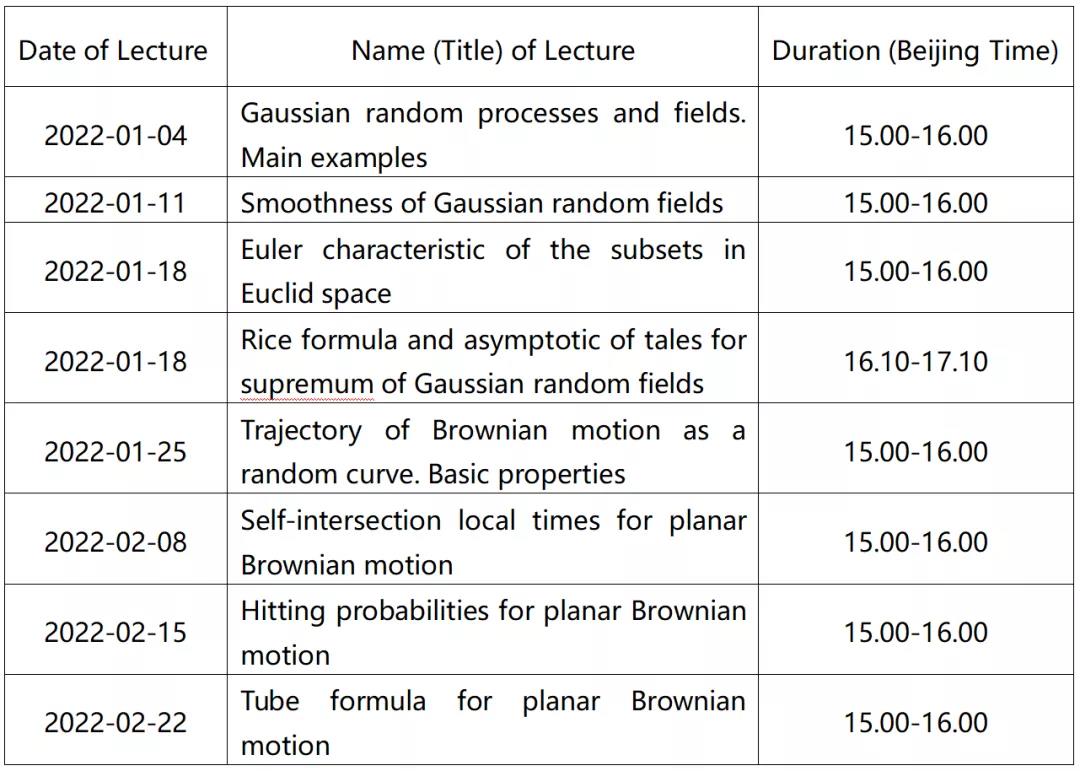
Lecture 1: Gaussian random processes and fields. Main examples
In this lecture we give the definition of Gaussian random processes and fields. Also, we will give some examples to understand this.
Lecture 2: Smoothness of Gaussian random fields
In this lecture we discuss the smoothness of Gaussian random fields, and we can get some properties on Gaussian random fields.
Lecture 3: Euler characteristic of the subsets in Euclid space
In this lecture we give the definition of Euler characteristic which in defined on Euclid space, it is topological invariant.
Lecture 4: Rice formula and asymptotic of tales for supremum of Gaussian random fields
In this lecture we give the Rice formula and discuss the asymptotic of tales for supremum of Gaussian random fields.
Lecture 5: Trajectory of Brownian motion as a random curve. Basic properties
In this lecture we discuss the trajectory of Brownian motion and give some basic properties about this.
Lecture 6: Self-intersection local times for planar Brownian motion
In this lecture we discuss the investigation of the local times of self-intersection as the most important geometric characteristics for planar Brownian motion.
Lecture 7: Hitting probabilities for planar Brownian motion
In this lecture we discuss the hitting probabilities and how we use this to describe the trajectory of planar Brownian motion.
Lecture 8: Tube formula for planar Brownian motion
In this lecture we give the Tube formula for planar Brownian motion, and use it to help us learn the trajectory of Brownian motion.

 

Bio: Prof. Andrey Dorogovtsev is a corresponding member of the National Academy of Sciences of Ukraine and the Head of the Department of the Theory of Random Processes, Institute of Mathematics, National Academy of Sciences of Ukraine. He mainly works on the theory of probability and its related fields. Prof. Dorogovtsev is a leading specialist in the study of probability theory in Ukraine and the Ukrainian principal investigator in several joint scientific programs between Ukraine and Germany, Ukraine and Russia. Also, he is the editor of many scientific journals, including "Theory of Stochastic Processes" and "Ukraine Mathematical Journal".

 

Knot theory

Speaker: Vassily Olegovich MANTUROV (Moscow Institute of Physics and Technology)

Time: Janurary 6, 2022 - January 30, 2022

Location: Tencent Meeting

          Room Number: 614-9548-1864

          Password: 372633

Abstract:

Lecture 1. Reidemeister moves. Colouring invariants, and the linking number.
2022/01/06 18:00-19:00 (Beijing time)
Abstract: We will introduce diagrams of knots and links. We use the diagrams to build two invariants of links: Coloring invariant and the linking number.
 

Lecture 2. The Kauffman bracket, the Jones polynomial.
2022/01/09 18:00-19:00 (Beijing time)
Abstract: We will use Kauffman bracket to prove that Jones polynomial is a link invariant.We will compute some examples and prove the Kauffman-Murasugi-Thistlethwaite Theorem.
 

Lecture 3.  Fundamental group. The knot group.
2022/01/13 18:00-19:00 (Beijing time)
Abstract: We will define a famous link invariant: the fundamental group of the knot complements. We will show that this group is not trivial if the knot is not trivial.
 

Lecture 4. The knot Quandle is a complete knot invariant.
2022/01/16 18:00-19:00 (Beijing time)
Abstract: Matveev and Joyce defined a knot invariant, the knot quandle.  This is a complete invariant. We can obtain many invariants from the knot quandle, for example, the knot group and coloring invariant.
 

Lecture 5.  The braid groups
2022/01/20 18:00-19:00 (Beijing time)
Abstract: We define the braid group. The action of the braid group on Aut(F_{n}) is complete.We will also discuss Markov Theorem and Alexander Theorem.
 

Lecture 6.  The Alexander polynomial.
2022/01/23 18:00-19:00 (Beijing time)
Abstract: We will introduce several ways to define the Alexander polynomial.
 

Lecture 7.  Vassiliev invariant.
2022/01/27 18:00-19:00 (Beijing time)
Abstract: We will introduce the Vassiliev invariant and prove that “polynomial” invariant are all come from the Vassiliev invariant.
 

Lecture 8.  Khovanov homology.
2022/01/30 18:00-19:00 (Beijing time)
Abstract: Khovanov homology is a categorification of the Jones polynomial, in the sense that its Euler characteristic is the Jones polynomial.

 

Bio: Professor Vassily Olegovich Manturov is from Moscow Institute of Physics and Technology. His research interest is low dimensional topology and knot theory.  He has published more than 150 papers and got more than 1500 citations. He got "Professor of RAS" in 2016 and he is one of the Managing Editors of the "Journal of Knot Theory and Its Ramifications". He has published many books, for instance, 《Parity in knot theory and graph-links. Contemporary Mathematics. Fundamental Directions》,《Low-dimensional Topology and Combinatorial Group Theory》,《Virtual Knots. The State of the Art》 and 《Knot Theory》. He held many international conferences, such as "4-th Russian China Russia-China on Knot theory and Related topics" and three International Conferences in the Mathematical Institute (Oberwolfach) on knot theory and low-dimensional topology".

 

 

 

 

 

 

O-operators on Lie infinity algebras

Speaker：Joana Nunes da Costa（University of Coimbra - Portugal）

Time：2021.12.03 16:00-18.00

Location：Zoom

              ID：862 062 0549

              Password：2021

ZOOM meeting：https://us02web.zoom.us/j/8620620549?pwd=NUhCMWpuTG9Od1RMNjhEdkdwTzlsUT09  

Abstract: We define O-operators on a Lie infinity algebra E with respect to an action of E on another Lie infinity algebra. We characterize these operators as Maurer-Cartan elements of a certain Lie infinity algebra obtained by Voronov's higher derived brackets construction. We determine the Lie infinity algebra that controls the deformation of O-operators with respect to a fixed action.

Bio：Joana Nunes da Costa is a professor of University of Coimbra, Portugal. She mainly works on Poisson geometry and mathematical physics.

 

The Lang-Trotter Conjecture and the Hardy-Littlewood Conjecture

Speaker：Hourong Qin（Nankai University）

Time：2021.11.26  09:00-11.00 

Location：Meeting Tencent

                 Meeting ID：662 784 567

Meeting：https://meeting.tencent.com/dm/qloVYRjPFHvl

Abstract:

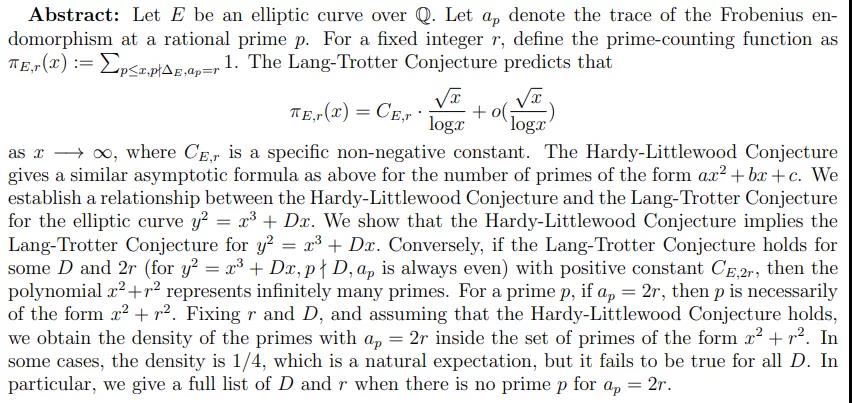
Bio： 秦厚荣教授现任南京大学数学系系主任，江苏国家应用数学中心主任，中国数学会常务理事，中国科学-数学编委，江苏省数学学会第十届，十一届理事长，第十二届监事会主席。1999年获得国家杰出青年基金，2004年受聘教育部长江学者计划特聘教授，首批入选国家“百千万人才计划”（2004年），享受国务院特殊津贴。他的研究方向主要是代数数论和代数K理论。他在同余数这一历史悠久问题上的研究上取得了重要成果；在数域的Tame核、Tate核方面做出了原创性工作，引发了大量后续工作；解决了田野，Browkin等人的多个猜想；在著名的椭圆曲线Anomalous素数的Mazur猜想以及Lang-Trotter猜想的研究中取得了突破。他在J. Reine Angew Math., P. London Math. Soc., Math. Ann. 等国际著名刊物上发表了数十篇论文，研究结果在国际同行中产生了广泛而积极的影响，被国外同行称为“秦的方法”，多次在高水平国际学术会议上作大会邀请报告。

Quantum GIM of N-fold affinization and quantum toroidal algebra

 

Speaker：Yun Gao（York University）

Time：2021.11.19  09:00-11.00 

Location：Zoom

                 Meeting ID：862 062 0549

                 Password：2021

ZOOM meeting：https://us02web.zoom.us/j/8620620549?pwd=NUhCMWpuTG9Od1RMNjhEdkdwTzlsUT09

Abstract: Generalized intersection matrix (GIM) Lie algebras were introduced by Slodowy in the study of elliptic singularity. GIM Lie algebras of N-fold affinization were studied by Berman-Moody, Benkart-Zelmanov and among others. In this talk we will talk about quantum GIM algebras of N-fold affinization and quantum toroidal algebras.

Bio： 郜云教授是加拿大York大学教授，德国洪堡学者。主要研究方向是无穷维李(超)代数、量子群和表示理论。在高维仿射李代数研究领域做出了重要工作。他已在国际一流数学杂志上发表论文50余篇，其中包括两本美国数学会专著(Memoirs of American athematical Society 1997和2002)。

 

Embedding of Loday algebras into Rota-Baxter algebras

Speaker：Vsevolod Gubarev（Sobolev Institute of Mathematics，Novosibirsk State University）

Time：2021.11.12 15:00-17:00 

Location：Zoom

                 Meeting ID：862 062 0549

                 Password：2021

ZOOM meeting：https://us02web.zoom.us/j/8620620549?pwd=NUhCMWpuTG9Od1RMNjhEdkdwTzlsUT09

Abstract：The classical Poincare–Birkhoff–Witt states that every Lie algebra injectively embeds into its universal enveloping associative algebra and this enveloping algebra in some sense does not depend on the Lie product (PBW-property). It is known that every Rota–Baxter algebra of weight 0/1 gives rise to a prealgebra/postalgebra. In 2013, it was proved that every pre- or postalgebra injectively embeds into appropriate Rota–Baxter algebra of weight 0 or 1 respectively. We study the structure and the PBW-property of the universal enveloping Rota–Baxter algebra of a pre- and post-Lie algebra.

Bio：Vsevolod Gubarev ，Senior researcher in Sobolev Institute of Mathematics and senior teacher in Novosibirsk State University (both in Novosibirsk, Russia). Area of interest: ring theory.  

 

Rota-Baxter operators and non-skew-symmetric solutions of the classical Yang-Baxter equation on quadratic Lie algebras

Speaker：Maxim Goncharov (Sobolev Institute of Mathematics，Novosibirsk State University)

Time：2021.11.5 15:00-17:00 

Location：Zoom

                 Meeting ID：862 062 0549

                 Password：2021

ZOOM meeting：https://us02web.zoom.us/j/8620620549?pwd=NUhCMWpuTG9Od1RMNjhEdkdwTzlsUT09

Abstract：Given a quadratic Lie algebra, it is well-known that skew-symmetric solutions of the classical Yang-Baxter equation are in one-to-one correspondence with skew-symmetric Rota-Baxter operators of weight zero. In this talk, we will study connections between Rota-Baxter operators of non-zero weight and non-skew-symmetric solutions of the classical Yang-Baxter equation on finite-dimensional quadratic Lie algebras. Particular attention will be given to the case of simple finite-dimensional Lie algebras.

Bio：Maxim Goncharov, Ph.D., Senior research fellow in Sobolev Institute of Mathematics, Associate Professor at Novosibirsk State University.

 

Revisiting and extending Poisson-Nijenhuis structures

 

Speaker：Henrique Bursztyn (Instituto Nacional de Matemática Pura e Aplicada)

Time：2021.10.22 08:00-09:00

Location：Zoom

                 Meeting ID：862 062 0549

                 Password：2021

ZOOM meeting：https://us02web.zoom.us/j/8620620549?pwd=NUhCMWpuTG9Od1RMNjhEdkdwTzlsUT09

Abstract：Poisson-Nijenhuis structures arise in various contexts, such as the theory of integrable systems and Poisson-Lie theory. I will revisit this notion from a new perspective and show how it can be extended to the realm of Dirac structures. I also hope to mention applications to integration problems. The talk is based on joint work with T. Drummond and C. Netto.

Bio：Henrique Bursztyn is a professor of Instituto Nacional de Matemática Pura e Aplicada (IMPA), Brazil. His research interest includes Poisson geomety, Dirac structures, Lie groupoids, Lie algebroids, deformation quantization and mathematical physics. He published more than 70 papers in high level journals, such as Duke Math. J,  J. Reine Angew. Math.,  Compos. Math.,  Comm. Math. Phys.,  Int. Math. Res. Not. IMRN, Adv. Math.  

Renormalization of quasisymmetric functions

Speaker：Li Guo (Rutgers University-Newark)

Time：2021.10.15 09:00

Location：Zoom

                 Meeting ID：862 062 0549

                 Password：2021

ZOOM meeting：https://us02web.zoom.us/j/8620620549?pwd=NUhCMWpuTG9Od1RMNjhEdkdwTzlsUT09

 

Abstract：The Hopf algebra of quasisymmetric functions (QSym) has played a central role in algebraic combinatorics and has broad applications. A natural linear basis of QSym is the set of monomial quasisymmetric functions defined by compositions, that is, vectors of positive integers. Extending such a definition for weak compositions, that is, vectors of nonnegative integers, leads to divergent expressions. This difficulty was addressed by a formal regularization in a previous work with Jean-Yves Thibon and Houyi Yu. Here we apply the method of renormalization in the spirit of Connes and Kreimer and realize weak composition quasisymmetric functions as power series. The resulting Hopf algebra has the Hopf algebra of quasisymmetric functions as both a Hopf subalgebra and a Hopf quotient algebra. It also gives a realization of free commutative Rota-Baxter algebra on one generator by weak quasisymmetric functions and thus addresses a question raise by Rota many years ago. This is a joint work with Houyi Yu and Bin Zhang. 

Bio：  郭锂，美国罗格斯大学纽瓦克分校教授。郭锂博士于兰州大学获学士学位，于武汉大学获硕士学位，于华盛顿大学获博士学位，并在俄亥俄州立大学、普林斯顿高等研究院和佐治亚州大学作博士后。郭锂博士的数论工作为怀尔斯证明费马大定理的文章所引用，并将重整化这一物理方法应用于数学研究。他近年来推动Rota-Baxter代数及相关数学和数学物理的研究，应邀为美国数学会在“What Is”栏目中介绍Rota-Baxter代数，并出版这个领域的第一部专著。研究涉及结合代数，李代数，Hopf代数，operad，数论，组合，计算数学，量子场论和可积系统等广泛领域.

 

Title：Koszul duality: old and new

Speaker：Andrey Lazarev(University of Lancaster)

Time：2021.10.8 15:00

Location：Zoom

                 Meeting ID：862 062 0549

                 Password：2021

ZOOM meeting：https://us02web.zoom.us/j/8620620549?pwd=NUhCMWpuTG9Od1RMNjhEdkdwTzlsUT09

 

Abstract：Koszul duality is a phenomenon occurring in homological algebra and neighbouring fields, such as rational homotopy theory, representation theory, algebraic geometry, operads and operadic algebras. In this talk I will outline a modern approach to deformation theory based on Koszul duality and explain how it can be globalized.  

 

Bio：Andrey Lazarev is a professor of pure mathematics in the University of Lancaster. His recent research deals with homotopy theory of differential graded algebras and categories, derived categories and higher phenomena. It has applications in rational homotopy theory, theory of operads and operadic algebras, algebraic topology and pure algebra. Andrey Lazarev is the Managing Editor of the journal《Bulletin of the London Mathematical Society》,as well as a Member of the Editorial Board of  the journal《Higher_Structures》.